You probably already know how probability works for rolling a die.
But did you know why Dungeons & Dragons likes to roll 4 dice, and remove the lowest roll, to generate optimal ability scores for a character?
Did you know that you're 20x as likely to roll an 18 with this method than to roll a 3?
Let's find out why below.

Calculating the probability of rolling any one number on a single die is simple.
You probably learned it in elementary school.
All you have to do is take the number of times your outcome occurs, and divide by the number of possible outcomes.
So, if you wanted to know the odds of rolling a 3 on a single six-sided die, you'd divide 1 (the number of times 3 shows up on the die) by 6 (the number of possible numbers you could roll.) This gives you a 1/6, or 16.7% probability of rolling a 3.
Since each number occurs exactly once on the die, each has an exactly equal probability of being rolled--1 out of 6.

If you roll two dice (that is, 2d6, as we say in the tabletop gaming industry), your results will be a little different.
As you can see in the graph above, you're much more likely to get a 7 than you are to get a 2 or a 12.
Why is that?
Well, suppose we were to make a table of all the possible outcomes from the two dice. It would look like this:
| First Die | Second Die | Total |
| 1 | 1 | 2 |
| 1 | 2 | 3 |
| 1 | 3 | 4 |
| 1 | 4 | 5 |
| 1 | 5 | 6 |
| 1 | 6 | 7 |
| 2 | 1 | 3 |
| 2 | 2 | 4 |
| 2 | 3 | 5 |
| 2 | 4 | 6 |
| 2 | 5 | 7 |
| 2 | 6 | 8 |
| 3 | 1 | 4 |
| 3 | 2 | 5 |
| 3 | 3 | 6 |
| 3 | 4 | 7 |
| 3 | 5 | 8 |
| 3 | 6 | 9 |
| 4 | 1 | 5 |
| 4 | 2 | 6 |
| 4 | 3 | 7 |
| 4 | 4 | 8 |
| 4 | 5 | 9 |
| 4 | 6 | 10 |
| 5 | 1 | 6 |
| 5 | 2 | 7 |
| 5 | 3 | 8 |
| 5 | 4 | 9 |
| 5 | 5 | 10 |
| 5 | 6 | 11 |
| 6 | 1 | 7 |
| 6 | 2 | 8 |
| 6 | 3 | 9 |
| 6 | 4 | 10 |
| 6 | 5 | 11 |
| 6 | 6 | 12 |
If you look over the results, you might notice that some totals appear more often than others.
For example, 9 can happen 4 times from rolling two dice. You can’t get it by rolling 3+6, 4+5, 5+4, or 6+3.
So, the probability of getting a 9 is 4/36, because there are 4 ways to get it out of 36 possible combinations.
In decimal form, that’s a probability of 11.11%.
The number 3, on the other hand, only occurs twice in the table.
Thats because you can only roll a 3 two ways: 1+2 and 2+1.
This gives you a probability of 2/36, or a 5.56% chance.
The difference in the probability of rolling different numbers with two dice plays a central role in board games like Settlers of Cataan, where you get a big advantage for owning a resource tile with a 7, 8, or 9 on it, but on a resource tile with a 2, 3, 11, or 12 may have you waiting the whole game to collect the resource even once.
Rolling multiple dice follows a normal distribution, which is also called a bell curve because of how it’s shaped.
The more dice you roll, the smoother the curve is.
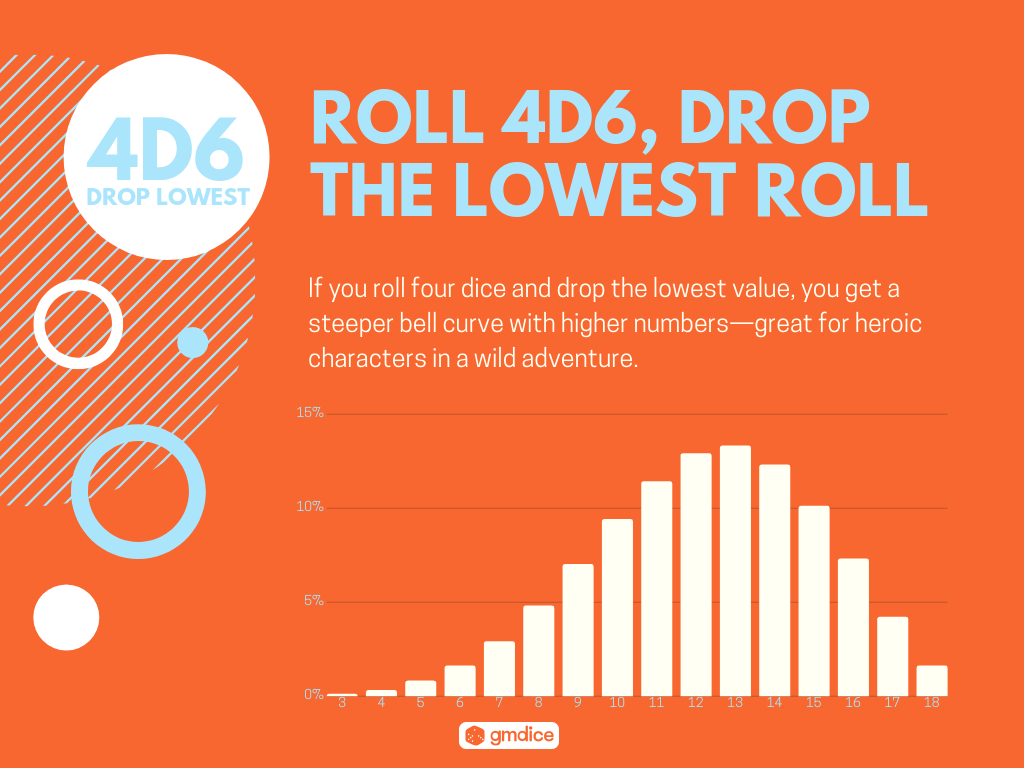
For Dungeons & Dragons, a common way to generate your character attributes (like strength, dexterity, and intelligence) is to roll 4d6, and drop the lowest roll.
In DnD, high attribute scores make your character powerful, and low attribute scores make your character weak.
The game is most fun when characters are about medium powerful, and it’s really not much fun if you have an abysmally low score like 3 or 4.
So, the game designers have players roll four dice to get a good bell curve and have mostly medium scores.
Then, they included the rule to drop the lowest die from the results, in order to skew the distribution a little to the right.
So, your scores will mostly be in the middle range, but you’re much more likely to have a very high score of 18 (a 1.62% chance) than a very low score of 3 (a 0.08% chance). That’s a difference of 20.25x, to be precise.
Of course, if you run a little more chaotic on the alignment scale and your Dungeon Master isn’t looking, I’ll bet your chances of getting an 18 are even higher than that.